¶ Stepper Motors
Check the drivers page to read about matching your stepper motors to the correct drivers.
The original design of PrintNC started out using Nema 17 motors.
Although adequate, it was quickly realized that Nema 23 motors were better suited to this application.
¶ Open Loop or Closed Loop?
What is the difference?
- Open loop stepper motors are instructed to move by the software/stepper motor driver and it is assumed that they have moved as instructed as there is no feedback. All the information is one way from the driver to the stepper motor. If a movement is too fast or there is too much force preventing the motor from moving, the controller will not know and the part being machined will not come out as expected.
- Closed loop stepper motors feedback their state to the controller. Therefore, if the motor skips in anyway, the controller knows to send more steps to make up for the loss. It usually does so by having some form of encoder attached to the stepper that counts the rotations.
There are other advantages of closed loop stepper motors over open loop, such as:
- Improved torque characteristics.
- Improved power/torque output curve.
- Higher running speeds.
- Better control of acceleration and deceleration.
Typical cost for a stepper motor + driver for an open loop system is somewhere in the region of $40-50 USD. Typical costs for a closed loop stepper motor + driver is somewhere in the region of $100+.
¶ Choosing a stepper motor
¶ Number of wires (unipolar/bipolar)
Simplest to connect are 4-wire motors. Inside the stepper motor are two coils, each coil having a wire connected to each end. The wire and coil pairs are called a phase. The 4 wires map to the 4 output pins of each stepper driver.
With 6-wire stepper motors, there are still 2 coils, but each coil has a centre tap, effectively cutting the coil in half if needed. This creates an extra wire for each coil. You can choose to run them in half-coil, by leaving two end-wires unconnected, or full coil mode by leaving the centre wires unconnected.
8-wire stepper have 4 coils, so with two wires per coil, this makes 8 wires. You can run an 8-wire stepper in half-coil (with only 2 coils connected) or full-coil mode, and in full-coil mode you can choose to wire the coils in series or in parallel.
¶ Rated current
This is the maximum current you may pass through both windings at the same time. The maximum current through one winding (which is what really matters when using microstepping) is rarely quoted and will be a little higher. However, even with one winding driven at the quoted rated current, the motor will get very hot. So the usual practice is to set the motor current to no more than about 85% of the rated current. Therefore, to get maximum torque out of your motors without overheating them, you should choose motors with a current rating no more than 25% higher than the recommended maximum stepper driver current of your driver.
¶ Holding torque
This is the maximum torque that the motor can provide with both windings energised at full current before it starts jumping steps. The holding torque with one winding energised at the rated current is about 1/sqrt(2) times that. The torque is proportional to current (except at very low currents), so for example if you set the drivers to 85% of the motor rated current, then the maximum torque will be 85% * 0.707 = 60% of the specified holding torque.
Torque is produced when the rotor angle is different from the ideal angle that corresponds to the current in its windings. When a stepper motor is accelerating, it has to produce torque to overcome its own rotor inertia and the mass of the load it is driving. In order to produce this torque, the rotor angle must lag the ideal angle. In turn, the load will lag the position commanded by the firmware.
You will sometimes see it written that microstepping reduces torque. What this really means is that when the lag angle is assumed to be equal to the angle corresponding to one microstep (because you want the position to be accurate to within one microstep), higher microstepping implies a smaller lag angle, hence lower torque. The torque per unit lag angle (which is what really matters) does not reduce with increased microstepping. To put it another way, sending the motor a single 1/16 microstep results in exactly the same phase currents (and therefore the same forces) as sending it two 1/32 microsteps, or four 1/64 microsteps, and so on.
¶ Step angle
There are two common step angles: 0.9 and 1.8 degrees per full step, corresponding to 400 and 200 steps/revolution.
Aside from the obvious difference in step angle:
- 0.9deg motors have slightly lower holding torque than similar 1.8deg motors from the same manufacturer
- However, to produce a given torque, the lag angle needed by a 0.9deg motor is slightly more than half the lag angle of a similar 1.8deg motor. Or to put it another way, at small lag angles a 0.9deg motor has nearly twice as much torque as a 1.8deg motor for the same lag angle.
- At a given rotation speed, a 0.9deg motor produces twice as much inductive back emf as a 1.8deg motor. So you generally need to use 24V power to achieve high speeds with 0.9deg motors.
- 0.9deg motors need step pulses to be delivered to the drivers at twice the rate of 1.8deg motors. If you use high microstepping then the speed could be limited by the rate at which the electronics can generate step pulses.
¶ Inductance
The inductance of the motor affects how fast the stepper motor driver can drive the motor before the torque drops off. If we temporarily ignore the back emf due to rotation (see later) and the rated motor voltage is much less than the driver supply voltage, then the maximum revs/second before torque drops off is:
revs_per_second = (2 * supply_voltage)/(steps_per_rev * pi * inductance * current)
If the motor is driving a GT2 belt via a pulley, this gives the maximum speed in mm/sec as:
speed = (4 * pulley_teeth * supply_voltage)/(steps_per_rev * pi * inductance * current)
Example: a 1.8deg/step (i.e. 200 steps/rev) motor with 4mH inductance run at 1.5A using a 12V supply, and driving a GT2 belt with 20 tooth pulley would start losing torque at about 250mm/sec.
In practice the torque will drop off sooner than this because of the back emf caused by motion, and because the above doesn't allow for the winding resistance. Low inductance motors also have low back emf due to rotation.
¶ Resistance and rated voltage
These are simply the resistance per phase, and the voltage drop across each phase when the motor is stationary and the phase is passing its rated current (which is the produce of the resistance and the rated current). These are unimportant, except that the rated voltage should be well below the power supply voltage to the stepper drivers.
¶ Back emf due to rotation
When a stepper motor rotates it produces a back emf. At the ideal zero lag angle, this is 90 degrees out of phase with the driving voltage, and in phase with the back emf due to inductance. When the motor is producing maximum torque and is on the verge of skipping a step, it is in phase with the current.
Back emf due to rotation is not normally specified on the data sheet, but we can estimate it from this formula:
approximate_peak_back_emf_due_to_rotation = sqrt(2) * pi * rated_holding_torque * revs_per_second / rated_current
The formula assumes that the holding torque is specified with both phases energised at the rated current. If it is specified with only one phase energised, replace the sqrt(2) by 2.
Example: consider a 200 step motor driving a carriage via a 20 tooth pulley and GT2 belt. That's 40mm movement per rev. To achieve a speed of 200mm/sec we need 5 revs/sec. If we use a motor with 0.55Nm holding torque when both phases are driven at 1.68A, the peak back emf due to rotation is 1.414 * 3.142 * 0.55 * 5/1.68 = 7.3V.
¶ Identifying the stepper motor phases
Here are two ways you can pair the stepper motor wires into phases:
- Use a multimeter. There should be a few ohms resistance between two wires that belong to the same phase, and no continuity between wires that belong to different phases.
- With the motor wires not connected to anything, spin the spindle between your fingers. Short two of the wires together, then spin the spindle again. If it is much harder to spin than before, those two wires belong to the same phase. Otherwise, try again with a different pair of wires shorted together.
¶ Motor Operating Temperature
Stepper motors are designed to run hot. Typically, motors are rated to operate in ambient environments up to 50°C and operate with up to an 80°C temperature rise. In other words, if your environment is 25°C, they can run at 105°C within specifications. You will want to turn the current if using the 3D printed motor mounts as they may soften while running your motors at full capacity.
¶
Frequently Asked Questions - PrintNC Stepper Motors
¶
¶ Q - Is a 600W 48V Meanwell PSU ( turned down) Overkill for 4 x 4.2 Amp stepper motors?
gerrg: No. I think that's probably getting pretty close to the limit actually. Might actually be a little under spec'd. (4*4.2A)*42V = 705.6W
Kopfsalat: Thank you. I might go parallel then.
gerrg: Or go cisco 1300W (the Cisco Catalyst Switch power supply used by many PrintNC community members). That would be enough
Logan: You can calculate your motor current at 2/3rds rating. So if they're 4.2A x 4 x 2/3 that's fine on a 600W power supply at 45V. I don't think they'll turn down to 42V. Cisco is nice because it's way overspecced, costs about the same as a meanwell 600W, and also has 12 and 5V outputs.
¶
¶ Q - Is there much spare capacity on the stock kit PSU from the AliExpress PrintNC store when run with the 3A kit motors?
For the standard Meanwell 350W PSU and 3A motors in the kit from the Three Design PrintNC Store on AliExpress the math would be:
3 Amps x 4 Motors x .66 X 36 V = 288W
288W / 350W = ~82.5% of PSU capacity. So the PSU is optimized for these motors and runs in at an efficient utilization.
¶
¶ Q - What are the specifications for the AliExpress PrintNC store 3A motors?
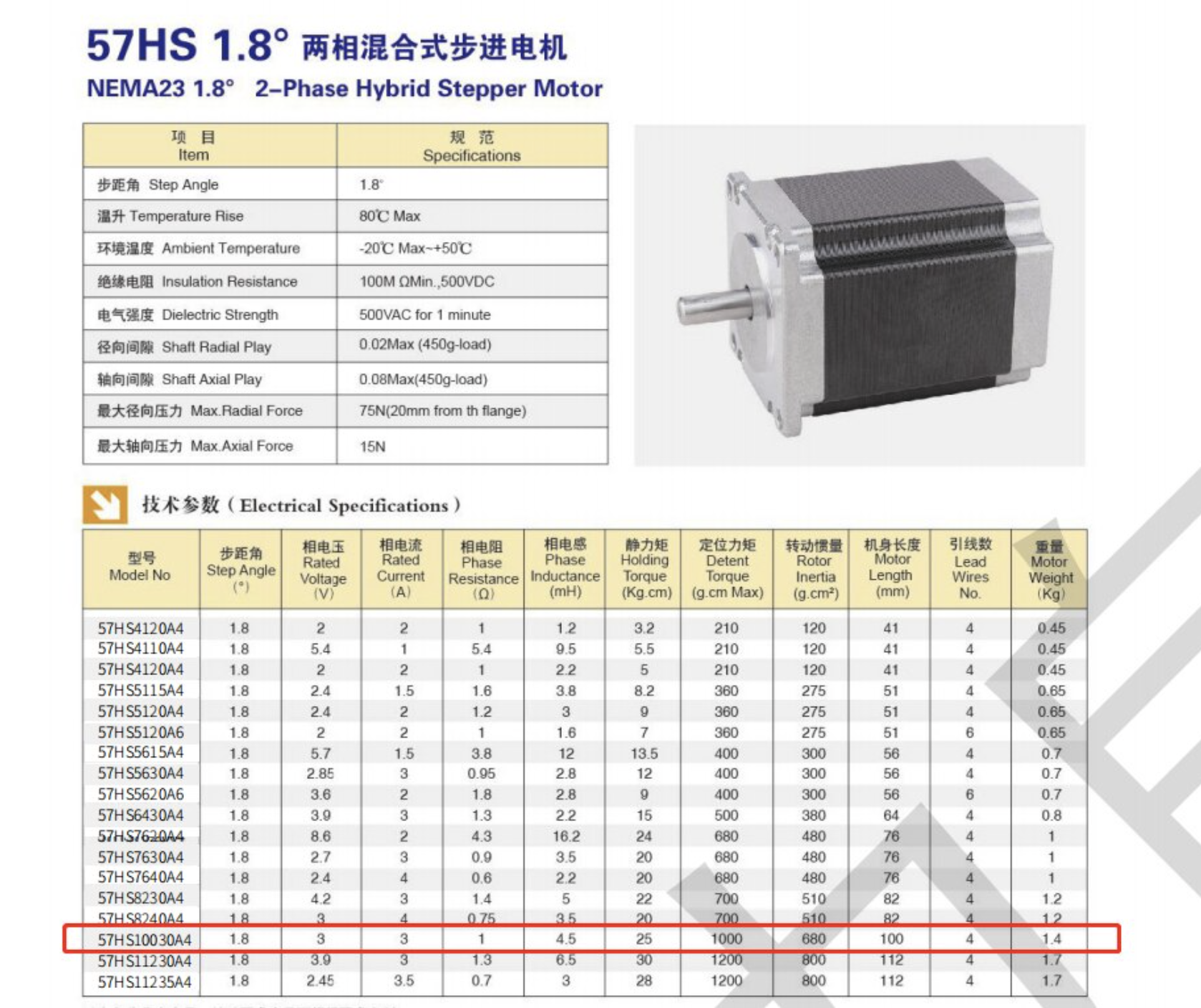
¶
¶ Q - What do flashing green LEDs mean on the stepper drivers?
Noot: I have the same problem. Squealing steppers sometimes and blinky green. I power my whole box off/on u til it goes away, and seems to work
For me, it appears to be caused by the signal lines from the BoB - unplugging them stops it
My "I haven't put a lot of thought or testing into it" theory is that it's related to grounding and/or noise
Once it's fixed (via trial and error), I've never had a problem until either my PC restarts or I flip my control box switch
kotlikm: Yeah, I really think I have bad drivers. If I swap the cables from the ones that work to the ones that don't, they still just flash green.